 How to Use a Calculator | Tips, Tricks & Techniques for Numerical Tests
How to Use a Calculator | Tips, Tricks & Techniques for Numerical Tests
Improve Your performance on Numerical Reasoning Tests by Becoming a Calculator God
Why You Should Master Your Calculator
The main difficulty in numerical reasoning tests is not the math, rather the time constraints. Knowing how to perfectly solve a question will not help if your calculations are slow.
Understanding your calculator and using its functions efficiently will be a key factor in your test performance.
We get very specific about how to use your calculator in the most proficient way further down this page.
If you feel you're ready to jump into the practical (and complicated) stuff, go ahead and click here - otherwise keep reading and we'll ease you into it.
Is a Calculator Allowed?
It is crucial that you know whether you're allowed a calculator ahead of time and prepare accordingly. This information should be provided on your test announcement. If it is not, do not hesitate to contact the HR department and ask.
As a rule of thumb, there are 3 types of tests with different approaches towards calculators:
- Calculation Tests: Calculation tests focus on your ability to perform basic calculations, mostly in the four operations. As such, no calculator is usually allowed in these tests. (Learn more)
- Cognitive Ability Tests: Here your ability to solve a large number of simple mathematical questions in a short period of time is put to the test. These tests also do not permit calculators. (Learn more)
- Numerical Reasoning Tests: Lastly, these traditional tests assess your ability to solve problems and draw conclusion from numerical data. As calculation is not considered the main measured ability of this test, calculators are sometimes allowed (Learn more).
Types of Calculators
There are 2 types of calculators: 1) simple and 2) scientific.

A simple calculator enables very basic functions such as:
- Addition (+), subtraction (-), multiplication (x) and division (÷)
- Percentage calculation
- 1 memory item (see below)
Meanwhile, a scientific calculator has a much broader range of options, including square roots and exponentials, logarithms, brackets etc.
Which Calculator is Allowed?
Most numerical reasoning tests only allow the use of simple calculators – but double check with your HR department. Sometimes, the definition of which calculators are allowed is based on their range of functions, e.g. four operations, percentage and square root.
Note: If a scientific calculator is allowed, the test provider will usually publish a list of allowed models.
Calculating Techniques
1) Using the memory in simple calculators
Although a pocket calculator allows for only the most basic of functions, it can save at least one number in its memory which can be of use for the next calculation. This can be done in several easy steps:
- View the number you wish to save
- Click on M+. The number is now saved to the calculator’s memory
- Pressing the MRC button (memory recall) will place the saved number onto the screen, allowing immediate usage for your next calculation
Let’s assume that we saved the number 12 to memory. We then begin a new calculation by pressing the number 36, and then pressing the division sign.
Finally we press the MRC button, which will place the number 12 after the divisor, giving us the equivalent calculation of 36/12. Pressing the equal sign will give us the correct result of 3.
Alternatively, we could have stored the number 36, and recalled it from memory at the beginning of the calculation.
Remember, a double click on the MRC button will clear the memory of any saved numbers.
In addition, we can add or subtract from the value stored in the memory directly by clicking on the M+ or M- buttons.
For example: Assuming we store 10 into the memory, we should:
a) Select the number 15
b) Press M- the calculator will subtract 15 from the value saved in the memory, giving us -5
c) Store that number in its place in the memory.
Similarly, pressing the M+ button when a number is displayed will add that number to the one stored in the memory.
2) Create a calculation plan
When a question requires more than one calculation step, it is extremely important to think ahead and organise the operations.
This way, there would be minimum use of paper and maximum usage of the calculator, since you can perform all the calculations in a row.
Test writers are aware of this strategy and create questions that provide this shortcut. Thus, it’s important to use it, otherwise you may waste precious time.
Example 1 - Government Construction Contracts (%)
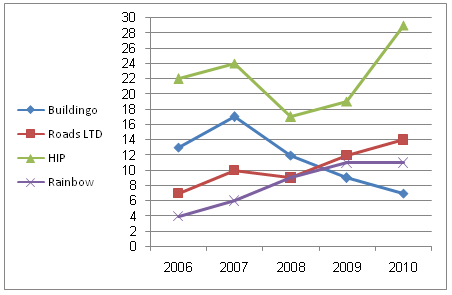
Of 400 contracts were offered for £22,200 each. In 2009, contract value increased by 7%, and there was a 25% decrease in the number of contracts.
How much more money than Buildingo did Roads do in 2009?
3) Look for repetitive operations
Always ask yourself - is there a specific operation that will be used more than once? If so, treat it like a common multiple, and leave it to the last step.
Consider this example:
Example 2
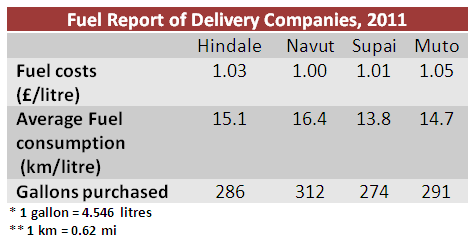
If a driver covered 500 miles with Navut's vehicle and 325 miles with Muto's, how many gallons did he end up using in total?
Final Tips
1. Practise quickly – efficient calculation work is an important part of success in the test.
2. Use your calculator for everything. If you're allowed a calculator, use it for even the most basic drills. It's faster, more accurate and no one will know that you've previously typed something into your calculator.
3. Bring a spare calculator with you (if you can). You don't want to be left high and dry if your calculator malfunctions during the test.


