 Numerical Reasoning Test Tips 2025 ▷ Improve Your Test Performance Fast
Numerical Reasoning Test Tips 2025 ▷ Improve Your Test Performance Fast
Get the Best Score on Your Numerical Test with Expert Techniques, Tricks & Formulas
How to Pass Your Numerical Reasoning Test
First thing you have to do is BREATHE, it's going to be okay. We've got you.
After you finish reading this guide you'll have a concrete understanding of the unique challenge this test presents and what you can do to get the score you want.
Read on to:
✓ Learn and apply our experts' strategies and methods for efficient question solving
✓ Learn easy ways to make shorter, faster, more accurate calculations
✓ Improve your abilities with our expert breakdown of example questions & answers
Test Anxiety & Mental Overload: How to Overcome Panic
When most people encounter a numerical reasoning question, they are overloaded by visual, numerical and textual data. The typical reaction is panic. The thought is they must fully understand every detail at once.
This causes people to feel as if they are facing insanely ‘difficult’ or ‘complicated’ questions. However, in most cases that is simply not the case. So remember to breath.
When solving a numerical reasoning question, address only what's relevant to the question. Don't try to attack an entire army of numbers and words at once. Instead, try to be strategic and take on one problem or variable at a time. It is our mission to teach you how.
A few things worth noting before we dive in:
1) Time is everything: There is no complex math in numerical reasoning tests. The difficulty lies in the short 45-75 seconds solving time given per question.
Most of the strategies you'll see on this page will help you shorten your solving time.
2) Estimation is key: Frequently, numerical reasoning tests are presented in multiple-choice. Therefore, estimation is key and an answer close enough is good enough!
3) Strengthening your mental muscles is a MUST: Forget about ‘you either have it, or you don't’. More than any other type of aptitude test, numerical reasoning crucially depends on training and practice.
You train, you get better - it's simple maths.
The format of multiple-choice questions combined with rigorous time constraints gives an advantage for those who practise seriously.

Tackling Numerical Reasoning Questions: The JobTestPrep Method
This is our personal 'world-famous' method to completely destroy most numerical reasoning questions.
It consists of 4 stages:
- Detect – what is the question asking
- Observe – separate the relevant from the irrelevant information
- Focus – focus on the relevant information and perform the necessary calculations
- Eliminate and Solve – use estimations and quick calculation methods to eliminate incorrect answers and solve the question
Let's take a look at an example:
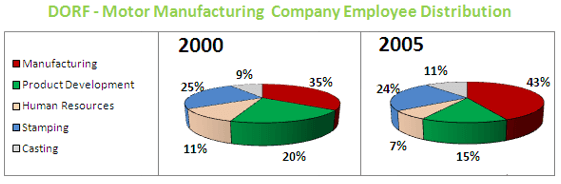
In the year 2000, the Product Development department constituted 400 employees.
How many employees worked for the Stamping department in that year?
A. 400
B. 450
C. 500
D. 550
Let's break the solving process by implementing the 4 stages of 'DOFE':
When we're asking about the number of employees in the Stamping department in 2000.
The relevant information is:
- The data from the year 2000.
- The number of employees in the product development department
- The number of employees in the stamping department
Thus, the question turns to:
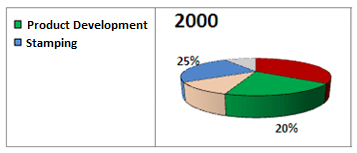
Quite a difference, isn't it?
Let's set the information we have in a table:
| Product Development | Stamping | |
| Percentage of Employees | 20% | 25% |
| Number of Employees | 400 | ? |
Since the calculation is very straightforward, we will not eliminate and look for the solution:
Using ratios:
(400/20) = (?/25)
? = 20 x 25 = 500
The number of employees in the stamping department is:
C (500).
Let's try another example:
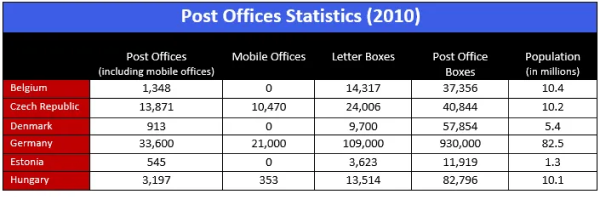
In Hungary, each person received 12 letters per month on average.
How many letters a month did people who don’t own a post office box receive in total if no two post office boxes are owned by the same person, and those who own post office boxes always get their post delivered to their post office boxes?
A. 182,198,000
B. 121,037,832
C. 142,936,000
D. 120,206,448
Try using our 4 stages again:
We're asked to find all the people in Hungary who DO NOT get mail by a post office box (POB), and multiply it by the number of letters.
The relevant information is:
- The total number of people in Hungary
- The number of people who DO get their mail by post office boxes
- The number of letters each person receives per month
Thus, the question turns to:
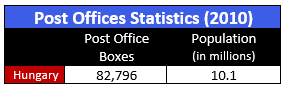
Number of letters per month: 12.
Now let’s break this whole lot of verbiage, intended to confuse you, to what it basically means:
... if no two post office boxes are owned by the same person, and those who own post office boxes always get their post delivered to their post office boxes?
No two post office boxes are owned by the same person:
- Number of people who own POBs = Number of POBs.
Those who own post office boxes always get their post delivered to their post office boxes:
- All mail received in POBs in Hungary = Number of POBs x Number of letters.
Now when we know all this, let's set a calculation plan:
[(All People in Hungary) -
(People Who Have POBs)] x
(Number of Letters per Month) =<
Again, we have a complete drill to just plug numbers into, so let’s do it:
[10,100,000 – 82,796] x 12 = 120,206,448
Thus, the correct answer is C (120,206,448).
You can get access to full-length mock tests and many more practice drills tailored to your exact test, by employer or test name.
Don't waste time practising generic materials that may or may not look anything like your real test. We research, compile and verify practice questions based on actual tests.

Don't Lose Time. Complete the Preparation You Started.
Improve your score within just a few days - with test prep specifically tailored after the test employers use.
Solving Techniques When You Can't Use a Calculator
Facing a test without a calculator is like facing a dragon without a sword. For such a frightening occasion we'd like to equip you with mathematical techniques used while solving problems calculator-less. Good luck!
Note: The methods presented here may not be the most intuitive ones, but oftentimes they are easier and shorter than straightforward calculations. As with any other solving method, experiment with them and see if they suit you personally.
1) Multiplication by 5
When you need to multiply a number by 5, divide it first by 2 and then multiply by 10.
Example:
168 x 5 = ?
The correct answer is: 840.
168 X 5 = (168 /2) x 10 = 84 x 10 = 840.
2) Use the Last Digit for Multiplication
Looking at the last digit of a multiplication can help you get to the correct answer without calculating.
Example:
123 x 429 = ?
A) 50,222
B) 52,767
C) 63,448
D) 55,409
The correct answer is: B.
Multiplying the last two digits of the exercise:
3x9 = 27, so the last digit of the result must be 7.
It's clear that the answer is: B (52,767).
3) Estimate Compound Growth Without a Calculator (Advanced Technique)
Use first-order and second-order elements to closely estimate growth/decrease.
Example:
You put £1,500 in the bank on the beginning of 2010. How much will you have by 2020 if the interest rate is 5%?
A) £1,750.25
B) £2,225
C) £2,355.60
D) £2,443.20
The correct answer is: D.
The formula for calculating compound growth is:
Final=Initial∙(1+i)N
Where:
i = growth rate (in our case – 5% = 0.05)
N = number of periods (in our case – 10)
Final=1500∙(1.05)10
It is evident that this calculation is quite long to do without a calculator. Therefore, we will use the following trick:
1. Calculate the first-order-approximation (FOA):
FOA=1+i∙N
FOA = 1 + 0.05 x 10 = 1.5
1st estimate: 1,500 x 1.5 = 2,250
2. Check if the estimation is close enough to reach the answer. In our case, answers C and D are rather close to the estimated answer. Thus, we will have to move on to step 3:
3. Calculate the second-order approximation (SOA) and add it to the result:
SOA=(N∙(N−1))2∙i2
SOA = [(10 x 9)/2] x 0.052 = 45 x 0.0025 = 0.1125
2nd estimate = 2,250 + 0.1125 x 1500 = 2,250 + 168.75 (you could very well estimate at 165 here, too) = 2,418.75
Thus, it becomes clear that the correct answer is D (£2,443.20).
Note: Since the approximations are always added, your approximate answer will always be lower than the real result.
We Help Over 70,000 People Every Year Improve Their Score - Fast

Don't Lose Time. Complete the Preparation You Started.
Improve your score within just a few days - with test prep specifically tailored after the test employers use.
Expert Tricks to Help You Avoid Unnecessary Calculations
The biggest problem that many face during numerical reasoning tests is the time limit.
Learning how to draw conclusion quickly enables you to answer more questions accurately and confidently.
1) Ignore multiplication factors
If you multiply and then divide by the same number, it can be ignored altogether.
Example:
A family's monthly petrol expense in year 1 is £250. What will be the percentage change of their annual petrol expense in year 2 if their monthly expense is £180?
To thoroughly calculate, we'd begin:
Annual expense in year 1: £250 x 12 = £3,000
Annual expense in year 2: £180 x 12 = £2,160
The change in percentage:
Change = [(3,000 – 2,160)/(3,000)]x100% = 28%
To calculate quickly, we'd begin:
Notice that in order to change the monthly expense to annual expense, we multiply by 12 and then divide by it:
Change = [(250x12– 180x12)/(250x12)]
Which is basically the same as:
Change = [(250-180)/(250)]x100% = 28%.
2) The '-1' percentage calculation
If you're looking for a change in percentage, take the ratio values and subtract one.
Example:
Looking at the question in the previous example, we could have just written:
Change = (180/250)-1 = 0.72-1 = -0.28
That means a decrease of 28%.
Did you notice we did not multiply by 100%? That is also a small tweak that can save you quite some time when accumulated.
Remember to convert decimals into percentages, move the period twice to the right:
0.28 → 28%
0.05 → 5%
2.36% → 236%
3) Create a calculation template
This might seem like a long process at first, but if you practise to perfection, it will save you valuable calculation time.
Create a framework in which you enter all your calculations, so eventually, you calculate only once.
Example:
Government Construction Contracts (%)
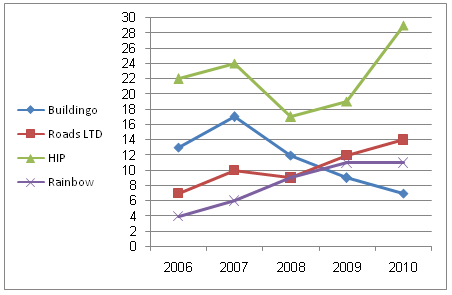
In 2008, a total of 400 contracts were offered for £22,200 each. In 2009, contract value increased by 7%. In addition, there was a 25% decrease in the number of contracts.
How much more money than Buildingo did Roads do in 2009?
A. 192,543
B. 217,386
C. 321,654
D. 423,964
E. 213,786
The correct answer is E (213,786).
1. Create a template. Since the question asks, ‘How much more …’, it would look like:
Answer = IncomeRoads - IncomeBuildingo
2. Break each part of the template into smaller parts:
Income = NC * PC
NC = TNC * Share(%)
Where:
TNC = Total Number of Contracts
NC = Number of company's Contracts
PC = Price per Contract
Share = Company's share of the contracts (from the graph)
So the final template will look as follows:
Answer = (TNC * Share * PC)Roads - (TNC * Share * PC)Buildingo
3. Identify common multipliers to minimize the template
- We know that the price per contract PC is similar in all cases:
PC = £22,200 x 1.07 = £23,754. - We know that the total number of contracts TNC is 400 x 0.75 = 300.
- The only difference between Roads and Buildingo is their share:
Buildingo has a share of 9%, Roads has a share of 12%.
4. Rearrange, plug in numbers and calculate once.
Now, after rearranging the numbers, our template will look like:
Answer = (300 ⋅ 23,754) x (0.12 - 0.09) = 213,786
Ace Your Job Search with a Custom Prep Kit
Job hunting doesn't have to be stressful.
Prepare smarter and ace your interviews faster with our Premium Membership.



General Tips for Numerical Reasoning
Numerical aptitude tests rely on basic mathematical concepts that can easily be reviewed and memorised. This includes the four operations (+, -, x, /) upon which further concepts are applied, like percentages, averages and ratios.
To succeed, you must master these basic concepts while drilling down into the complex questions of graphs and tables, number series and word problems.
Don't try to solve the entire question nor understand the load of information presented before you. Focus on the question, and apply only the relevant, useful data.
Since most numerical tests use some sort of graphs and tables to present information, you should get used to reading this type of data:
- Financial sections of daily newspapers and, of course, practice questions and sample tests are the best way to familiarise yourself with possible types of data presentation.
- Pay attention to units and scales. It is very common to find questions where the information is presented in millions when the question actually refers to thousands.
- Pay attention for comments, asterisks, etc. They are easily missed and are often crucial to solve the questions.
- Use the orientation test: After a few seconds of scanning the data, place your finger somewhere on the data area and explain that piece of data.
Know in advance if you're allowed a calculator and prepare accordingly.
No Calculator Allowed: Improve your basic numeracy calculations, as they might be the difference between failure and success.
Allowed to use a Calculator: Learn to skillfully know your device. Using your calculator wisely can become a substantial time saver. For instance, people who are not accustomed to using a calculator tend to write each result instead of leaving it on the calculator's screen for the next calculation.


