 Get Thorough Practice for Your Numerical Reasoning Test & Pass With a High Score
Get Thorough Practice for Your Numerical Reasoning Test & Pass With a High Score
Master numerical aptitude tests top employers use in banking, engineering, civil service, and finance.
Evaluate your current skill level with our sample questions covering all critical test components with complete solutions and expert guidance:
- Numerical drills for calculation speed
- Number series for pattern recognition
- Word problems for practical application
- Tables and graphs for data interpretation
Our Numerical Reasoning PrepPack™ is designed to transform beginners into experts with targeted practice materials.
Free Numerical Reasoning Test - Amp up Your Performance With Example Numerical Questions
Check out our Free Aptitude, Abstract Reasoning, Verbal Reasoning, Spatial Reasoning, and Critical Thinking Tests.
Numerical Drills
This section covers the fundamental numerical reasoning skills and competencies that underlie success on any numerical test: mental arithmetic, basic operations, ratios, percentages, algebra, and more.
Numerical Reasoning > Numeracy Drills > Basic Maths
Basic Maths Sample Question
Choose the difficulty:
Ace Your Job Search with a Custom Prep Kit
Job hunting doesn't have to be stressful.
Prepare smarter and ace your interviews faster with our Premium Membership:

See What People Think About Our Membership
Numerical Reasoning > Numeracy Drills > Algebra
Algebra Sample Question
if

what is the value of y?
Answer
This numerical reasoning question has two important lessons: taking out the common factor and using the multiple-choice answers to your advantage.
To solve this problem, take out the common factor in the numerator and in the denominator in order to simplify the expression.
The result is:

You know that

thus

Multiply both sides by 2y and get 5=2y, which is reducible to y=2.5.
While this is a straightforward solution, taking a glance at the possible answers before solving can expedite the process even further. After taking out the common factor, a sharp eye could have noticed that the ratio between the numerator and the denominator is 2.5:y. This is easier to notice if you already know that 2.5 is one of the answers. Looking at the answers can be a helpful tool during numerical reasoning tests.
Numerical Reasoning > Numeracy Drills > Geometry
Geometry Sample Question

What is the area of a parallelogram with a base length of 8 cm and a height of 5 cm?
Answer
Geometry problems can often seem daunting at first, only because the solver is unaware of a certain basic rule that would clear it all up. In this case, you must realize that a parallelogram is simply a rectangle whose corner has been displaced. If you imagine cutting the polygon in the picture along the dashed line and moving the triangle to the right side, such that d and b are coinciding sides, you get a perfect rectangle.
We know the area of a rectangle is the product of the base and height, so parallelograms will work the same way: the area of a parallelogram equals the product of the length of the base and the corresponding height (i.e. the height that is perpendicular to the chosen base).
Substituting with the numbers from the question: 8 x 5 = 40.
Therefore, the area of the parallelogram is 40 cm2.
Tip: If your exam is a week or more away, we recommend giving basic numerical reasoning questions like these a good number of days. There is truly no substitute for having an intuitive understanding of the cogs and bolts that make maths tick. However, if you are pressed for time, start with more advanced maths practice tests and only go back to review topics that you notice yourself repeatedly struggling with.
The numerical reasoning questions you just answered sample three 'Numerical Drills' topics: Basic Maths (mental arithmetic), Algebra, and Geometry. These have been selected because they represent three pillars of maths that underpin numerical literacy and aptitude as a whole. On our Numerical Reasoning Tests PrepPack™, you will also be able to delve into four more 'Numerical Drills' topics: ratios, percentages, averages, and currency conversions.
The types of numerical questions featured in this section are more focused on revamping your numerical aptitude than simulating any real aptitude test. However, there are numerical reasoning exams that do, in fact, include questions like these. For example: SHL, CCAT, the Optiver Test, and Aon Cut-e's scales eql test. These are called Basic Numeracy Tests.
Pass Your Numerical Reasoning Test
Number Series Questions
This section covers number sequencing problems. These numerical questions call on you to discover the pattern of a sequence of numbers and fill in the blanks.
Numerical Reasoning > Number Series > Sample Question #1
Number Series Sample Question #1
Please choose one correct answer:
0.125 | 0.4 | 0.5 | 1.4 | 2 | 2.4 | ?
Answer
The odd terms (indicated in red) create an inner sequence that increases by x4 each step.
The correct answer is 8.
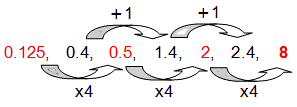
💡 TIP: It is common for one series to have two logical rules that alternate throughout. If you can't figure out how the items are connected, try breaking it into two logical rules: one for odd items and one for even items. A series with more terms is likelier to be one of these.
Numerical Reasoning > Number Series > Sample Question #2
Number Series Sample Question #2
Please choose one correct answer:
1 | 3 | 11 | 67 | ?
Answer
The correct answer is 4, as is explained here:
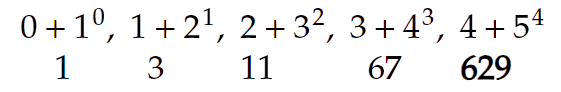
Each term is arrived at using a number: 'a'. The formula is a + (a+1)a. The number 'a' starts at zero and increases by 1 with each step.
A formal presentation of this concept:
a + (a+1)a, (a+1) + (a+2)a+1, … (a+n) + (a+n+1)a+n
💡 TIP: Series that require figuring out a formula are the hardest to solve, as they are unintuitive and tricky. A good approach to these questions is remembering that you are meant to solve them within a limited timeframe and without the help of a calculator. Hence, the formula has to be relatively simple. A multiplication between consecutive numbers, raising to a certain power, and making different calculations using a specific order of mathematical operations - are the common forms used. We recommend considering an elaborate formula (like the one in number series question #2) only after dismissing all other familiar patterns.
Specific numerical tests that feature number series questions include: the GSS Test, MMAT Test, CCAT, PI Cognitive Assessment, Cubiks Logiks Test, Suited Assessment, and Mettle Test.
Number series prove time and again to be the most dreaded form of numerical reasoning tests. This is most likely due to the fact that there is no protocol that can be safely followed to assuredly produce the correct result. Rather, the candidate has to wait for a "penny drop" moment, which they fear may never come.
While this all may be true, it is important to stress that as with other psychomteric tests - numerical instincts and intuition can and should be acquired. Practising before the exam can make a significant difference.
JobTestPrep's Numerical Reasoning Test Practice Centre
Use our guides to study for your numerical reasoning tests:
Word Problems
In this section, you will find numerical ability questions that are presented in the form of a story. The challenge is to intuit what mathematical formulas, operations, or expressions accurately reflect the problem.
Numerical Reasoning > Word Problems > Percentages
Percentages Sample Question
Due to an increase in taxes on electronic devices, the price of a 46” LED flat TV screen has increased to £845, which is a 30% increase from the original price. What was the original price of the TV prior to the increase?
Answer
In this numerical reasoning test question, the whole (100%) is the original price and is therefore our x.
A good way to tackle percentage problems is by writing down the given information in a table with two columns: real values and percentages, while leaving space for the data you don't have:

Then, you can use the “triangle trick” in order to calculate the missing data: Multiply along the diagonal and then divide by the remaining number.
Applying this method to the question at hand:

In order to find the missing data, multiply the numbers connected by the diagonal (the hypotenuse) and then divide by the number located on the remaining vertex:
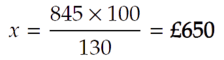
Another approach to this type of question requires an understanding of the relation between a given percentage and the proportion it represents (and vice versa). This relation is represented by the following formula:
(Total = the value of the 100%)
You can isolate the part you are interested in:
Total = (Value x 100) / %
And insert the data: Total = (845 x 100) / 130 = £650.
Numerical Reasoning > Word Problems > Travel #1
Travel #1 Sample Question
Richard and Grace planned to meet at a restaurant. Since it was a lovely day outside they decided to walk there. Richard started at point A, located 820 meters away from point B, which is where Grace began her walk. If Richard walks 6 times faster than Grace, what is the ratio between the respective distances covered?
Answer
The correct answer is 6:1.
The image below shows a model of the question. This model is accurate because we are told that Richard and Grace walked in the same direction.

If we call the restaurant C, we are essentially being asked to find the ratio AC:BC. We know that Richard walks six times faster than Grace and that they arrive at the same time.
The relationship between time, distance, and speed (or velocity) can be described thusly:

This means that as long as t (time) stays the same, as is the case here, the proportionate sizes of d (distance) and v (velocity) will stay the same. If one were to increase, the other would have to increase as well. To put this in mathematical terms, speed and distance are directly proportional.
Therefore, the ratio of walking speed between Richard and Grace must be the same as the ratio of distance walked. Therefore, Richard walks six times the distance that Grace walked:
XRichard : XGrace = 6v*t : v*t = 6 : 1
Numerical Reasoning > Word Problems > Travel #2
Travel #2 Sample Question
Dylan drove 66 miles in 55 minutes. How many minutes longer would he have had to drive if his speed had been 5 mph less?
Answer
The correct answer is 4.1.
We've already seen that the formula describing the relationship between velocity, time, and distance is

Another formulation of this same relationship is distance = velocity x time. We can use it to find Dylan's velocity. Since t (time) is expressed in minutes and we are looking for his velocity in miles per hour, we will first convert it into hours: 55 minutes (=55/60 hours). Now:
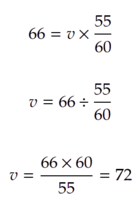
Therefore, Dylan’s velocity is 72 mph. Had it been 5 mph slower, it would have equalled 72 - 5 = 67 mph. In that case, the time it would have taken him to drive 66 miles is:

According to this, it would have taken Dylan 59.1 - 55 = 4.1 minutes longer.
Numerical Reasoning > Word Problems > Equations
Equations Sample Question
The owner of a liquor store offers a special deal on a certain wine, in which a pair of bottles are sold with a 30% discount on the second bottle. If the cost of one bottle for the owner is £2.5, and on selling 50 pairs with the aforementioned discount he made a £260 profit, what is the selling price of one bottle of wine on that sale?
Answer
The correct answer is £6.
Let the selling price of a bottle be S. The selling price of one pair of bottles after the discount would be:
S + (0.7 x S) = 1.7S
If the cost of one bottle for the owner is £2.5, then the profit from selling a pair of bottles is:
1.7S - (2 x 2.5) = 1.7S - 5
You are told that the owner made a £260 profit on selling 50 pairs of bottles, thus you can create the following equation:
(1.7S - 5) x 50 = 260
85S - 250 = 260
85S = 510
S=6
Thus, the selling price is £6.
💡 TIP: After finding the answer you can check yourself by plugging £6 as the selling price, and calculating the profit for 50 pairs.
Numerical Reasoning > Word Problems > Combinatorics
Combinatorics Sample Question
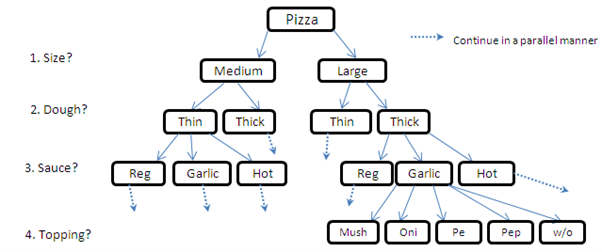
Juliana is not certain which pizza to order. She can have the medium or large-size pizza, with thin or thick dough; replace the regular with hot or garlic sauce; add one topping if she'd like: mushrooms, onions, peppers or pepperoni.
How many different pizzas can Juliana order?
Answer
The correct answer is 60.
In order to solve this numerical reasoning test problem, you must figure out the correct number of choices in each category. We have 4 categories:
Size – medium or large = a choice between 2 options.
Dough – thin or thick = a choice between 2 options.
Sauce – regular, hot or garlic = a choice between 3 options.
Topping - mushrooms, onions, peppers, pepperoni or without = a choice between 5 options.
The overall number of combinations equals the product of the number of options within each category: 2x2x3x5 = 60.
Another way to view this sort of problem is to sketch a flow chart:
💡 TIP: Word problems obscure the mathematical problem to be solved with stories and information, some of which is relevant and some of which isn't. There are several strategies for sifting through the information quickly. Most commonly: it is helpful to be an active reader. This means doing things while you read like highlighting or underscoring important information, or scribbling a diagram of the question on the margin. Another helpful tip is to read the bottom line first and then, when you know what to look for, read the entire question.
Ace every test that comes your way
three PrepPacks, 50% off, one smart membership. Start prepping now!”

Word problems in numerical reasoning tests are designed to evaluate your ability to see maths not just when they're in the form of a numerical problem, but also in day-to-day situations. One might call them "applied numerical ability questions".
Specific numerical reasoning tests that feature word problems include the CCAT, MMAT, Cubiks Logiks, and PI Cognitive Test.
Note: out numerical reasoning test practice pack is a one-size-fit-all product. It contains practice tests that prepare you for any real test. If you know the name of your test provider of company - look it up in the search box. The side menu contains the names of common numerical reasoning tests.
Tables & Graphs
The most common type of numerical reasoning tests question, here you will be asked to infer numerical data presented in table or graph form. This numerical ability test evaluates your data interpretation skill and ability to draw conclusions. Take note: the graphs get more complex as you go!
Numerical Reasoning > Tables & Graphs > Tables
Tables Sample Question
What was the revenue generated from Chianti sales?

Answer
Once we've sifted out the relevant information from the table, the question becomes a simple conversion between percentage and absolute numbers. We know that 13% of the 5550 Chianti bottles were defective. This means 87% were not. Using the formula presented higher up on this page, we can calculate that 87% of 5500 is equal to 0.87 x 5500, which is 4785.
Since each bottle of Chianti costs £22, the revenue generated was 4785 x 22 = 105,270.
💡 TIP: Keep your focus on the relevant details of the question you were asked. While tables can contain a lot of details, your question concerns only a small portion of them. Identify the information you need and analyze every aspect of it.
Numerical Reasoning > Tables & Graphs > Graphs
Graphs Sample Question
What proportion of both sites' April and May traffic was for Cuisine.net?

Answer
To calculate this, we add up the total page views of cuisine.net for April and May and divide this amount by the total hits of both sites for the same period.
Therefore, the calculation would be:
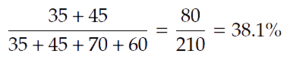
💡 TIP: While working with percentages, make sure you know which group is your whole. Are you calculating percentages out of the total amount, or out of a specific category?
Graphs, tables, and chart questions are the most common numerical questions because they bring together various different numerical abilities: basic maths, ratios, and percentages, a real-world context for the question, and attention to detail.
Questions of this type feature prominently on the Aon/cut-e numerical tests, SHL numerical tests, Talent Q, Saville Assessments, some CS Fast Stream Schemes, and Cubiks Logiks. Also, if you're interested in Abstract or Quantitative reasoning questions see the following UCAT practice test and UCAT test prep.
Congratulations!
If you made it this far, you have completed our free numerical reasoning practice test! How did you do?
Numerical reasoning tests are a complex field of testing and one that candidates often walk into not knowing what to expect or fearing that their full potential might not shine through to their prospective employers.
Practice is the key to gaining the confidence needed to stop relying on luck, and to be sure your full abilities are reflected in the test every time you take one.
JobTestPrep's Numerical PrepPack walks you through numerical drills, number series questions, word problems, and tables and graphs at a pace that lets you maximise your gain. It also includes access to video and PDF guides and tutorials with valuable tips and tricks.
If you are looking for more numerical test tips, you can find them in our video on the subject:
Numerical Reasoning Test Tips
How do numerical reasoning tests work?
Of the various aptitude tests, the numerical reasoning test is particularly feared and disliked. This is not very surprising. Most people haven't been excited to take a maths test since primary school, if that. Numerical ability quite simply doesn't come naturally to most people. (If you are reading this thinking: "What? I love a good maths test!" Congratulations. You are among the lucky few.)
So what is the key to success at numerical tests?
Well, at JobTestPrep, we believe practice tests are essential. This is because a numerical reasoning assessment isn't meant to ascertain your grasp of mathematical concepts. Rather, numerical tests tend to assess your numerical logic, ability to quickly interpret numerical data, answer questions naturally and swiftly, and perform calculations with ease. This is why most tests have a time limit.
These numerical skills can only be acquired by doing. Practice tests help you gain these abilities. Our free tests, and the numerical aptitude tests on our PrepPacks, feature example questions that have been meticulously selected to include all the skills needed to ace a real numerical reasoning test.
Here are some more tips for your numerical test:
- Take advantage of the multiple-choice format
As with most pre-employment psychometric tests, numerical reasoning tests usually allow you to choose from a number of options (A notorious exception is the SHL Interactive Numerical Reasoning Test). This opens up new options for reaching the correct answer, such as process of elimination. - Don't fear the time limit
The best way of dealing with strict time limits on a numerical test is by first skipping all the test questions you need more than a few seconds to think about. Only after going through the entire numerical test once and solving all the easy questions, you can get around to performing difficult numerical computations. This ensures you don't miss any easy questions by getting stuck early in the test. In fact, this advice works for most aptitude tests. not just numerical reasoning. - Sharpen your mathematical skills
Numerical ability tests take time and practice to ace. Take advantage of practice tests as much as you can. This will not only make you better at maths, but it will also give you the option of assessing where you stand every step of the way, so you know when you are ready for the real test.
Are you about to apply for a role in the finance industry?
Most major banking and consulting employers evaluate their applicants using various numerical tests, among others. Visit your potential employer's page to understand better the tests you are about to face, and start preparing today!
HSBC | UBS | Bain & Co | Macquarie | Morgan Stanley | Barclays | EIB | Deloitte | Deutsche Bank | KPMG | PWC | Lazard | EY | Nomura | BCG | BNP Paribas | Jefferies | Moelis & Co
More Free Practice
We at JobTestPrep find the assessment tests world highly diverse and fascinating. If you are looking to deepen your knowledge in the aptitude tests world, or you want some extra practice before your test, we've got you covered!
Check out these fantastic free practice tests (all are completely free):
Free Aptitude Test | Free Psychometric Test | Free Watson Glaser Test | Free SHL Test | Free Thomas GIA Test | Free Korn Ferry Test | Free UCAT Practice Test


